FME02 – Cap 02 – Ex. 79

Enunciado

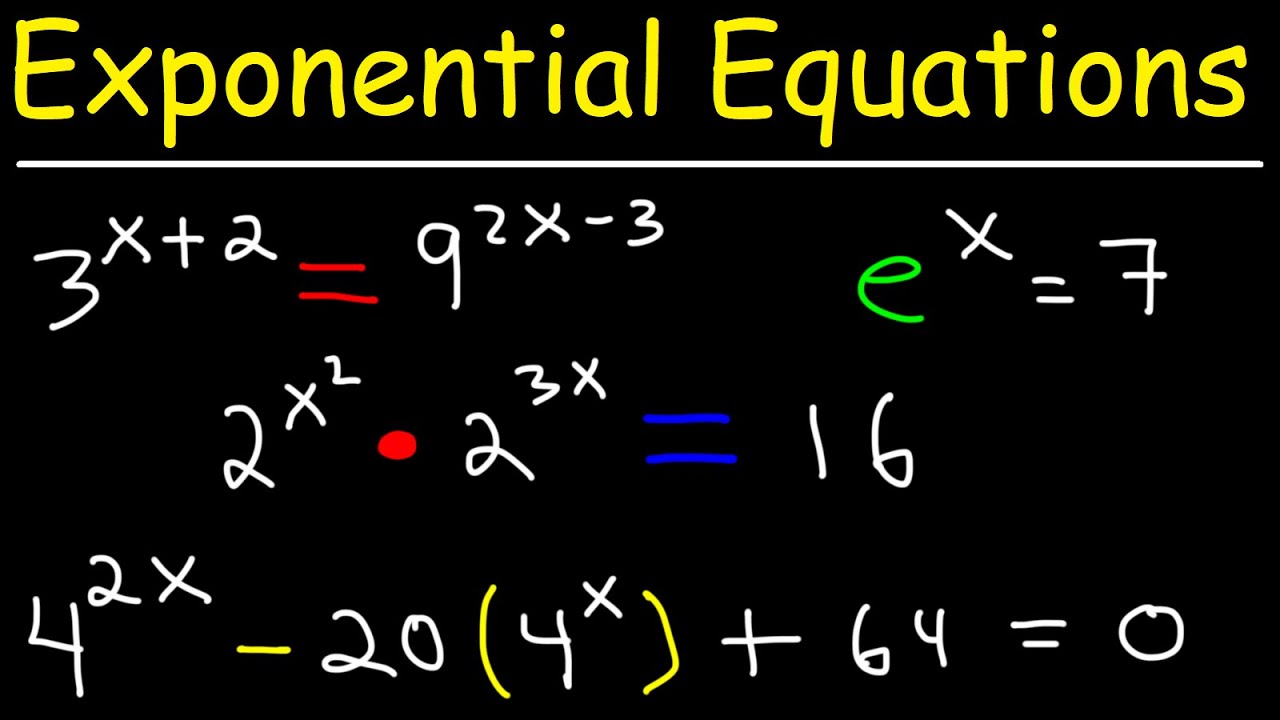
Resolva as seguintes equações exponenciais:

$3^{x-1} - 3^x + 3^{x+1} + 3^{x+2} = 306$
$5^{x-2} - 5^x + 5^{x+1} = 505$
$2^{3x} + 2^{3x+1} + 2^{3x+2} + 2^{3x+3} = 240$
$5^{4x-1} - 5^{4x} - 5^{4x+1} + 5^{4x+2} = 480$
$3 \cdot 2^x - 5 \cdot 2^{x+1} + 5 \cdot 2^{x+3} - 2^{x+5} = 2$
$2 \cdot 4^{x+2} - 5 \cdot 4^{x+1} - 3 \cdot 2^{2x+1} - 4^x = 20$

Entendimento

Exercício de aplicação direta das propriedades de exponenciais. O objetivo aqui é chegar a uma expressão na forma $base^{expoente_1} = base^{expoente_2}$ .

É um tipo de exercício relativamente simples, mas que exige muita atenção e uma boa dose de inciativa. Ao longo do processo de solução temos que ir verificando quais as melhores base e expoente a serem empregados. Sair fazendo contas apressadamente, em geral, não é o melhor caminho.

Resolução: item a.

3^{x-1} - 3^x + 3^{x+1} + 3^{x+2} = 306 \\ \dfrac{1}{3} \cdot 3^x - 3^x + 3 \cdot 3^x +3^2 \cdot 3^x =2 \cdot 3^2 \cdot 17 \\ \frac{(1 - 3 + 9 + 27)}{3} \cdot 3^x = 2 \cdot 3^2 \cdot 17 \Rightarrow \frac{34}{3} \cdot 3^x = 34 \cdot 3^2 \\ \frac{1}{3} \cdot 3^x = 3^2 \Rightarrow 3^x = 3^3 \Rightarrow x = 3

Resolução: item b.

5^{x-2} - 5^x + 5^{x+1} = 505 \\ \dfrac{1}{25} \cdot 5^x - 5^x + 5 \cdot 5^x = 5 \cdot 101 \\ \frac{(1 +100)}{25} \cdot 5^x = 5 \cdot 101 \Rightarrow 101 \cdot 5^x = 125 \cdot 101 \Rightarrow 5^x = 5^3 \\ x = 3

Resolução: item c.

2^{3x} + 2^{3x+1} + 2^{3x+2} + 2^{3x+3} = 240 \\ 2^{3x} + 2 \cdot 2^{3x} + 2^2 \cdot 2^{3x} + 2^3 \cdot 2^{3x} = 240 \\ (1 + 2 + 4 + 8) \cdot 2^{3x} = 240 \Rightarrow 15 \cdot 2^{3x} = 15 \cdot 16 \\ 2^{3x} = 2^4 \Rightarrow x = \dfrac{4}{3}

Resolução: item d.

5^{4x-1} - 5^{4x} - 5^{4x+1} + 5^{4x+2} = 480 \\ \dfrac{1}{5} \cdot 5^{4x} - 5^{4x} - 5 \cdot 5^{4x} + 5^2 \dot 5^{4x} = 480 \\ \dfrac{(1 + 5 \cdot 19)}{5} \cdot 5^{4x} = 480 \\ 96 \cdot 5^{4x} = 48 \cdot 10 \cdot 5 \Rightarrow 5^{4x} = 5 \cdot 5 \\ 4x = 2 \Rightarrow x = \dfrac{1}{2}

Resolução: item e.

3 \cdot 2^x - 5 \cdot 2^{x+1} + 5 \cdot 2^{x+3} - 2^{x+5} = 2 \\ 3 \cdot 2^x - 5 \cdot 2 \cdot 2^x + 5 \cdot 2^3 \cdot 2^x - 2^5 \cdot 2^x = 2 \\ (3 - 10 + 40 -32) \cdot 2^x = 2 \\ 2^x = 2 \Rightarrow x = 1

Resolução: item f.

2 \cdot 4^{x+2} - 5 \cdot 4^{x+1} - 3 \cdot 2^{2x+1} - 4^x = 20 \\ 2 \cdot 4^2 \cdot 4^x - 5 \cdot 4 \cdot 4^x - 3 \cdot 2 \cdot 2^{2x} - 4^x = 4 \cdot 5 \\ 32 \cdot 4^x - 20 \cdot 4^x - 6 \cdot 4^x - 4^x = 4 \cdot 5 \\ (32 - 20 - 6 - 1) \cdot 4^x = 4 \cdot 5 \\ 5 \cdot 4^x = 4 \cdot 5 \Rightarrow x = 1

Neste item é preciso um pouco de cuidado no tratamento do expoente de $2^{2x+1}$ .

Dificuldade: 2,12

Entendimento: 1,5
Conceitos: 2,0
Iniciativa: 2,5
Cálculos: 2,5